
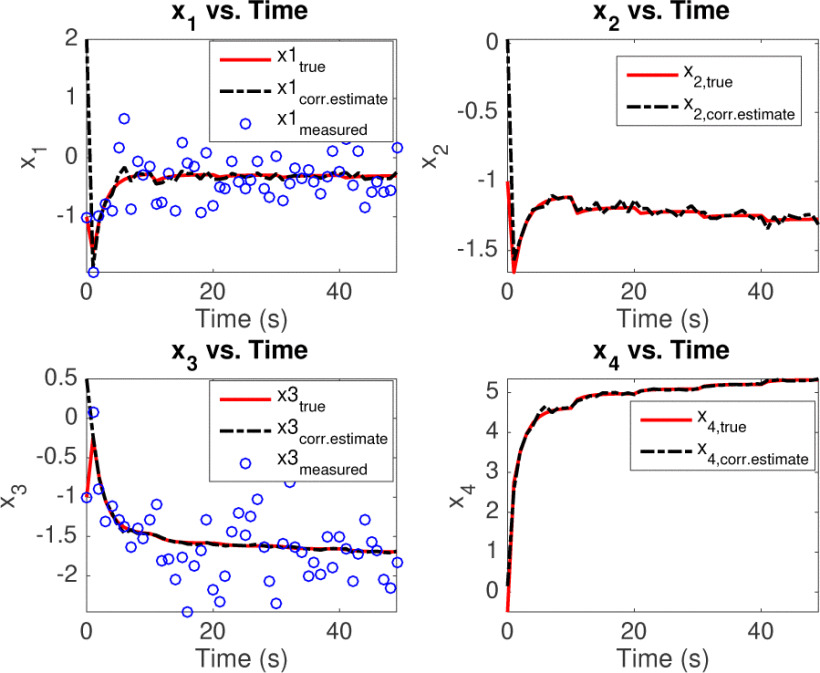

For a system of linear equations in two variables, we can determine both the type of system and the solution by graphing the system of equations on the same set of axes. The first step would be to choose one of the equations and solve it for either x or y. Graph a system of linear equations There are multiple methods of solving systems of linear equations.
It does not matter which equation you choose first, or which variable you solve for first the values for both variables will be the same.įor example, given the system of linear equations: The procedure is repeated by adding back. Setting up a system of equations from context example (pet weights) Setting up a system of linear equations example (weight and price) Interpreting points in context of graphs of systems. Systems of equations with graphing: exact & approximate solutions. The solution is then used in finding the second to last variable. Systems of equations with graphing: y7/5x-5 & 圓/5x-1. This method works by solving one of the linear equations for one of the variables, then substituting this value for the same variable in the other linear equation and solving for the other variable. This equation is then solved for the one unknown. Substitution will have you substitute one equation into the other elimination will have you add or subtract the equations to eliminate a variable graphing will have you sketch both curves to visually find the points of intersection. Follow these steps: Rearrange the equation so 'y' is on the left and everything else on the right. There are three ways to solve systems of linear equations: substitution, elimination, and graphing. To solve a system of linear equations without graphing, you can use the substitution method. The second graph above, being 'Case 2' in the middle column, shows two distinct lines that are parallel. Graph the 'equals' line, then shade in the correct area.


 0 kommentar(er)
0 kommentar(er)
